This paper was awarded in the II International Competition (1993/94) "First Step to Nobel Prize in Physics" and published in the competition proceedings (Acta Phys. Pol. A 88 Supplement, S-69 (1995)). The paper is reproduced here due to kind agreement of the Editorial Board of "Acta Physica Polonica A".

MAGNETIC SURFACE ANISOTROPY IN FERROMAGNETIC
THIN FILMS WITH TWO ROUGH SURFACES

M. REWIEŃSKI

Nicolaus Copernicus High School, Wały Piastowskie 6, 80-855 Gdańsk, Poland

Abstract

In this paper the magnetostatic energy in a ferromagnetic thin film with two imperfect, rough surfaces has been calculated. It has been shown that the surface roughness gives in some cases a considerable contribution to an effective perpendicular anisotropy. Within the model assumed it has been proved that the value of magnetostatic energy and, as a consequence, the anisotropy in a thin imperfect film does not depend on the relative location of the two rough surfaces.
PACS numbers: 75.70.-i

1. Introduction

Discovery of the phenomenon of perpendicular anisotropy has gained much interest in the past few years. It has opened new possibilities for memory applications. Ferromagnetic thin films have been widely investigated for use as a data storage medium in magneto-optic or magneto-recording systems [1, 2].

The perpendicular magnetic anisotropy is attributed to the interfacial surface anisotropy, which is a straightforward consequence of the fact that surface atoms are located in a different environment than the bulk ones. It has been found that at least three mechanisms give rise to the surface anisotropy. Those are the single-ion mechanism, dipole-dipole interaction and also the effect caused by the surface roughness.

The importance of the interfacial surface roughness in the phenomenon of magnetic anisotropy was suggested by Bruno [3]. In this paper we examine only the surface roughness, as a factor which contributes to the dipolar magnetic anisotropy. We derived formulas determining the magnetostatic energy and the surface anisotropy as functions of some geometrical parameters of an imperfect ferromagnetic thin film. The calculation of the magnetostatic energy and the surface anisotropy has been performed within the macroscopic theory of magnetism. (We have applied the continuous medium approximation.)

2. Model of surface roughness

In this paper we shall adopt the model of surface roughness proposed by Bruno [3]. The difference between the model of Bruno and the actual model used in the calculation is that we consider a ferromagnetic film having two rough surfaces and finite thickness t. The thickness t may be considered an average, i.e. the experimentally measured distance between surfaces of the film. The following parameters also characterize our probe: the average deviation $\sigma$ from the plane of the surface the average lateral size d of ``craters'' or ``terraces'', i.e. the flat areas on the surface, and the phase difference $\psi$ between ``craters'' on one surface and the other (cf. Fig. 1).

Fig.1. Representation of a thin film with surface roughness showing the characteristic parameters t, $\sigma$, d and $\psi$.

3. Calculation of magnetostatic energy

Calculation of the magnetostatic energy is based on the standard Fourier series method proposed first by Kittel [4]. The magnetostatic energy is given by the following expression:

$$E_{M}=\int -\frac{1}{2} \vec{M} \cdot \vec{H} {\rm d}V. \eqno(1)$$

We assume that the film shown in Fig. 1 is magnetized with the saturation magnetization $\vec{M}_{\rm s}$ normal to the surface. The examined film may be considered as a superposition of three magnetization distributions, as it is shown in Fig. 2. In the left upper corner of Fig. 2 there is a side view of the thin film. Below that the decomposition of the magnetized film into three parts is shown.

Fig.2. Decomposition of the magnetization distribution in a thin film with two rough surfaces.

Arrows indicate direction of magnetization of each specific region in space. A picture in the right upper corner of Fig. 2 shows the top and the bottom view of the film. The right column of Fig. 2 shows the top view of each of the component parts forming together our rough ferromagnetic film. The gray regions are ``terraces'' and the white ones are ``craters''. The signs + and - indicate the direction of magnetization in each region. Let us denote the magnetizations of the corresponding parts as $\vec{M}_{1}$, $\vec{M}_{2}$ and $\vec{M}_{3}$, demagnetizing fields as $\vec{H}_{1}$, $\vec{H}_{2}$ and $\vec{H}_{3}$, distributions of magnetic poles on surfaces of each probe as $\sigma_{1}$, $\sigma_{2}$ and $\sigma_{3}$ and, eventually, scalar potentials of demagnetizing fields as $\phi_{1}$, $\phi_{2}$ and $\phi_{3}$ correspondingly (Fig. 2). Equation (1) transforms now as follows:

$$E_{M}=\int -\frac{1}{2} \vec{H} \cdot \vec{M} {\rm d}V = \int... ... \vec{M}_{1}+\vec{M}_{2}+\vec{M}_{3} \right){\rm d}V. \eqno(2)$$

It is important to notice that

$$\int -\frac{1}{2} \vec{H}_{i} \cdot \vec{M}_{i}{\rm d}V=\int ... ...{i}\phi_{i}\vert _{z={\rm surface}2} \right) {\rm d}S. \eqno(3)$$

In accordance to Fig. 2 and making use of (3) we transform Eq. (2)

$$E_{M} = \frac{1}{2} \int_{0}^{2d} \int_{0}^{2d} \left(\sigma_... ...2}\vert _{z=t-2\sigma}-\sigma_{2} \phi_{2}\vert _{z=0} \right.$$

$$\qquad +\sigma_{3} \phi_{3}\vert _{z=t-2\sigma}-\sigma_{3} \... ... \phi_{3}\vert _{z=0}+\sigma_{3} \phi_{1}\vert _{z=t-2\sigma}$$

$$\qquad -\sigma_{3} \phi_{1}\vert _{z=0}+\sigma_{1} \phi_{2}\... ... \phi_{1}\vert _{z=t-2\sigma}-\sigma_{2} \phi_{1}\vert _{z=0}$$

$$\qquad \left. +\sigma_{2} \phi_{3}\vert _{z=t-2\sigma}-\sigma... ...a_{3} \phi_{2}\vert _{z=0}\right) {\rm d}x {\rm d}y. \eqno(4)$$

The function of distribution of magnetic poles $\sigma(x,y)$, which has a period 2d, may be expanded in a double Fourier series

$$\sigma(x,y)= \sum_{-\infty}^{\infty} \sum_{-\infty}^{\infty} ... ...ft(\frac{m\pi x}{d}+\frac{n\pi y}{d} \right) \right]. \eqno(5)$$

The Fourier coefficients C_mn are given by the expression

$$C_{mn}=\frac{1}{4\pi^{2}} \int_{0}^{2\pi} \int_{0}^{2\pi} \s... ...(x,y)\exp[-{\rm i}(m\xi+n\eta)]{\rm d}\xi {\rm d}\eta, \eqno(6)$$

$$\left(\xi={\pi x}/{d}, \eta={\pi y}/{d} \right).$$

In the same way we can expand the scalar potential $\phi(\xi,\eta)$ of the magnetic field $\vec{H}$

$$\phi(\xi,\eta) = \sum\sum \phi_{mn}\exp\left[ {\rm i}(m\xi+n\eta)\right] \exp(-P_{mn}z). \eqno(7)$$

The above potential satisfies Laplace's equation and the condition of continuity of the field on the surface of a probe: $\nabla^{2}\phi=0$, $\left. -\frac{\partial \phi}{\partial z} \right\vert _{z={\rm surface}}=4\pi \sigma(x,y)$. Therefore we obtain

$$\phi_{mn}=4\pi \frac{C_{mn}}{- P_{mn}},\quad P_{mn}=(m^{2}+n^{2})^{1/2}{\pi}/{d}, \quad (n,m \not= 0). \eqno(8)$$

We calculate the coefficients (6) for distributions of magnetic poles $\sigma_{1}$, $\sigma_{2}$ and $\sigma_{3}$ in each of the component parts of our probe. According to Fig. 2 the function $\sigma_{1}(x,y)$ is given by the following formula:

$$\sigma_{1}(x,y)\vert _{x,y \in[0,2d]} = \left\{\begin{array}{... ...) \times (0,d)] \cup[(0,d)\times (d,2d)] \end{array}. \right.$$

The results of the calculation are given below

$$C_{00}^{(1)}=\frac{M_{\rm s}}{2},\quad C_{m0}^{(1)}=C_{0n}^{(... ...= {-2M_{\rm s}}/{(\pi^{2}mn}),\quad(m,n - {\rm odd}). \eqno(9)$$

We can also write formulas for $\sigma_{2}$ and $\sigma_{3}$

$$\mbox{\hspace*{- 6mm}}\begin{array}{c} \sigma _{2}(x,y)\\ x,y... ...,2d-\psi) \times (d,2d-\psi)]] \end{array} \end{array} \right.$$

$$\mbox{\hspace*{-6mm}} \begin{array}{c} \sigma _{3}(x,y)\\ x,y... ...,d-\psi)\times (2d-\psi,2d)]. \end{array} \end{array} \right.$$

Analogically we obtain coefficients for the functions $\sigma_{2}$ and $\sigma_{3}$:

$$C_{00}^{(2)}=C_{m0}^{(2)}=C_{0n}^{(2)}=0,\quad C_{mn}^{(2)} =... ...c{2M_{\rm s}}{\pi^{2}mn}(1-\exp({\rm i}\delta(m+n))), \eqno(10)$$

$$C_{00}^{(3)}=\frac{M_{\rm s}}{2},\quad C_{m0}^{(3)}=C_{0n}^{(... ...frac{2M_{\rm s}}{\pi^{2}mn}\exp({\rm i}\delta(m+n)), \eqno(11)$$

where m, n are odd and $\delta={\pi \psi}/{d}$, cf. Fig. 1.
Substituting (9), (10) and (11) into expression (5) yields

$$\sigma_{1}=\frac{M_{\rm s}}{2}-\sum_{k=-\infty}^{\infty} \su... ...s}\exp({\rm i}((2k+1)\xi+(2l+1) \eta))}{\pi^{2}(2k+1)(2l+1)},$$

$$\mbox{\hspace*{-6mm}} \sigma_{2}=\sum_{k=-\infty}^{\infty} \... ...!+\!1)\xi\!+\!(2l\!+\!1)\eta))}{\pi^{2}(2k\!+\!1)(2l\!+\!1)},$$

$$\mbox{\hspace*{-6mm}} \sigma_{3}=\frac{M_{\rm s}}{2}+\sum_{k=... ...\!+\!1)\xi\!+\!(2l\!+\!1)\eta))}{\pi^{2}(2k\!+\!1)(2l\!+\!1)}.$$

We can also derive formulas for the potential (7) by making use of the expressions (9)-(11)

$$\phi_{1}=\frac{4\pi M_{\rm s}}{2} z-\sum_{k=-\infty}^{\infty}... ...{\infty}\frac{8\pi M_{\rm s}}{(- 1)\pi^{2}(2l+1)(2k+1)P_{kl}}$$

$$\qquad \times \exp({\rm i}((2k+1)\xi+(2l+1)\eta))\exp(-P_{kl}z),$$

$$\phi_{2}= \sum_{k=-\infty}^{\infty} \sum_{l=-\infty}^{\infty... ...rm i}\delta((2k+1)+(2l+1))))}{(- 1)\pi^{2}(2l+1)(2k+1)P_{kl}}$$

$$\qquad \times \exp({\rm i}((2k+1)\xi+(2l+1)\eta))\exp(-P_{kl}z),$$

$$\phi_{3}=\frac{4\pi M_{\rm s}}{2}(z+2\sigma)+\sum_{gk=-\inft... ...({\rm i}\delta((2k+1)+(2l+1)))}{(-1)\pi^{2}(2l+1)(2k+1)P_{kl}}$$

$$\qquad \times \exp({\rm i}((2k+1)\xi+(2l+1)\eta))\exp(- P_{kl}(z+2\sigma)),$$

where

$$P_{kl}=\frac{\pi}{d} \left[(2k+1)^{2}+(2l+1)^{2}\right]^{1/2}.$$

Now we already know all the potentials and functions of distributions of magnetic poles, so we are able to calculate the integral (4). As an example we shall calculate one component of the sum (4)

$$\frac{1}{2} \int_{0}^{2d} \int_{0}^{2d}(\sigma_{1}\phi_{1}\ve... ...0}^{2d}\int_{0}^{2d}4\pi \left( \frac{M_{\rm s}}{2}\right)^{2}t$$

$$-\frac{4\pi M_{\rm s}}{2}t \sum_{k=-\infty}^{\infty} \sum_{l... ...\rm s}\exp({\rm i}\pi/d((2k+1)x+(2l+1)y))}{\pi^{2}(2l+1)(2k+1)}$$

$$-\frac{M_{\rm s}}{2}\sum_{k=-\infty}^{\infty}\sum_{ l=-\infty... ...exp(- P_{kl}t)\!-\!1)}{(-1)\pi^{2}(2k\!+\!1)(2l\!+\!1)P _{kl}}$$

$$+\sum_{k=-\infty}^{\infty} \sum_{l=-\infty}^{\infty} \frac{2... ...rm s}\exp({\rm i}\pi/d((2k+1)x+(2l+1)y))}{\pi^{2}(2k+1)(2l+1)}$$

$$\times \sum_{k=-\infty}^{\infty}\sum_{l=-\infty}^{\infty}\fr... ...!1)}{(- 1)\pi^{2}(2k\!+\!1)(2l\!+\!1)P_{kl}}{\rm d}x{\rm d}y.$$

Let us note that $\int_{0}^{2d}\exp({\rm i}\pi nx/d){\rm d}x=0$ for $n \not= 0$. Then the above expression reduces to the following form:

$$\int_{0}^{2d} \int_{0}^{2d}(\sigma_{1}\phi_{1}\vert _{z=t} -... ...{2d}\int_{0}^{2d}4\pi \left( \frac{M_{\rm s}}{2} \right)^{2}t$$

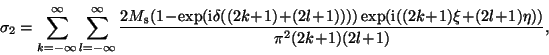
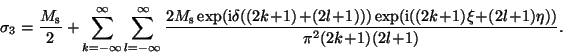
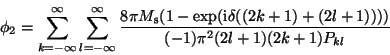
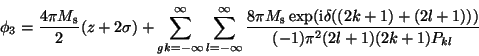
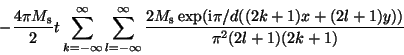
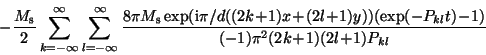
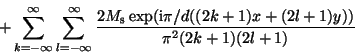
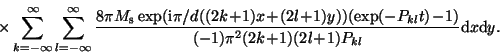
$$\qquad +\frac{16M_{\rm s}^{2}}{\pi^{3}}\sum_{k=-\infty}^{\inf... ...\exp(- P_{kl}t)]}{(2l+1)^{2}(2k+1)^{2}P_{kl}}{\rm d}x{\rm d}y.$$

In the same way we calculate all the other components of the integral (4). The result of derivation of the expression for magnetostatic energy is shown below

$$E_{M}^{\perp}=\frac{1}{2}4\pi M_{\rm s}^{2}(2d)^{2} \Big\{t-... ...g[\frac{((2k+1)^{2}+(2l+1)^{2})^{- 1/2}}{(2k+1)^{2}(2l+1)^{2}}$$

$$\quad\times (1-\exp(-P_{kl}t)+\exp(-P_{kl}(t-2\sigma)) -\exp(- P_{kl}2\sigma)$$

$$\quad +{\rm i}\sin(\delta((2k+1) +(2l+1)))(\exp(-P_{kl}t)-\exp(-P_{kl}2\sigma)))\big]\Big\}.$$

As the function $\sin(x)$ is odd and the sum shown above goes through both positive and negative numbers, the component ${\rm i}\sin(\delta((2l+1)+(2k+1)))(\cdot)$ does not make any contribution to the sum. Eventually we can write the equation for the magnetostatic energy with magnetization normal to the surface of the probe

$$E_{M}^{\perp}=\frac{1}{2}4\pi M_{\rm s}^{2}(2d)^{2}\Big\{ t-\sigma+\frac{4^{2}d}{\pi^{5}}$$

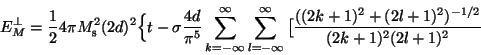
$$\qquad \times \sum_{k=0}^{\infty}\sum_{l=0}^{\infty} \left[\... ...k+1)^{2}(2l+1)^{2}((2k+1)^{2}+(2l+1)^{2})^{1/2}}\right] \Big\}$$

where $P_{kl}=\frac{\pi}{d}[(2k+1)^{2}+(2l+1)^{2}]^{1/2}.$ One of the most important features of the equation above is that it is not dependent on $\delta$. It means that the value of magnetostatic energy does not depend on relative location of ``craters'' between the upper surface and the lower surface.

Fig.3. Graph of the magnetostatic energy $E_{M}^{\perp}$ as a function of the average deviation $\sigma$ from the plane of the surface.

Fig.4. Graph of the function $f(\sigma)$.

In Fig. 3 the magnetostatic energy has been shown as a function of the average deviation $\sigma$ from the plane of a surface, for some chosen values of ratio t/d ( $\sigma \in[0,t/2)$, d=1). Figure 4 shows values of the function $f(\sigma)$ defined below, also for the chosen values of ratio t/d ( $\sigma \in[0,t/2)$, d=1)

$$f(\sigma)=\frac{4^{2}d}{\pi^{5}\sigma}\sum_{k=0}^{\infty} \s... ...{(2k+1)^{2} (2l+1)^{2}((2k+1)^{2}+(2l+1)^{2})^{1/2}} \right).$$

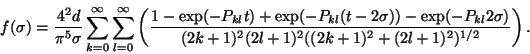
4. Surface anisotropy

As we already know the magnetostatic energy of a thin film with magnetization normal to the surface, we can easily derive the value of magnetostatic energy of this film with magnetization parallel to the plane of the surface. To do this we apply the sum rule presented by Yafet et al. [5]. By the symmetry reasons we have that $E_{M}^{x}=E_{M}^{y}=E_{M}^{\parallel}$ (the magnetostatic energy with magnetization parallel to the x axis equals the magnetostatic energy with magnetization parallel to the y axis). The energies E_M^x, E_M^y and E_M^z satisfy the following equation:

$$E_{M}^{x}+E_{M}^{y}+E_{M}^{z}=2E_{M}^{\parallel}+E_{M}^{\perp}=\frac{1}{2 } 4\pi M_{\rm s}^{2}St.$$

Therefore we get

$$E_{M}^{\parallel}=\frac{1}{4}4\pi M_{\rm s}^{2}St -\frac{1}{2} E_{M}^{\perp}.$$

We can write that

$$E_{M}^{\parallel}-E_{M}^{\perp}=\frac{1}{4}4\pi M_{\rm s}^{2}... ...s}^{2}St-\frac{3}{4}4\pi M_{s}^{2}S(t-\sigma+\sigma f(\sigma))$$

$$\qquad\qquad =\frac{3}{4}4\pi M_{\rm s}^{2}S\sigma(1-f(\sigma)) -\frac{1}{2}4\pi M_{\rm s}^{2}V.$$

In Fig. 5 the values of energy anisotropy $(E_{M}^{\parallel}- E_{M}^{\perp})/(2\pi M_{\rm s}^{2})$, as a function of the average deviation $\sigma$ from the plane of a surface, have been shown for some chosen values of ratio t/d ( $\sigma \in[0,t/2)$, d=1).

Fig.5. The difference between the magnetostatic energies $(E_{M}^{\parallel}-E_{M}^{\perp})/2\pi M_{\rm s}^{2}$ as a function of $\sigma$.

Fig.6. The surface anisotropy constant K_S as a function of the average deviation $\sigma$ from the plane of a surface.

The difference between magnetostatic energies with magnetization parallel and normal to the plane of the surface can be written in the following form:

$$E_{M}^{\parallel}-E_{M}^{\perp}=VK_{V}+2SK_{S},$$

where K_V is the dipolar volume anisotropy constant and K_S -- the surface anisotropy constant. Therefore we obtain

$$K_{S}=\frac{3}{2}\pi M_{\rm s}^{2} \sigma (1-f(\sigma)).\eqno(12)$$

Figure 6 shows the surface anisotropy constant (12) as a function of the average deviation $\sigma$ from the plane of a surface, also for some chosen values of ratio t/d ( $\sigma \in[0,t/2)$, d=1).

5. Conclusion

In this paper we have derived formula describing magnetostatic energy in a ferromagnetic thin film with two rough surfaces magnetized with saturation magnetization $M_{\rm s}$ normal to the plane of the surface. The value of magnetostatic energy depends on the average distance between the surfaces. Still it has been found that it does not depend on the parameter $\psi$, i.e. the phase difference between ``craters'' on the higher surface and ``craters'' on the lower surface. In other words, relative location of the two surfaces does not influence the value of magnetostatic energy. Also the values of energy anisotropy have been calculated. In case of our thin film the difference between magnetostatic energies ( $E_{M}^{\parallel}-E_{M}^{\perp}$) remains a negative number within the range of parameters t, d and $\sigma$ used. It means that the easy direction magnetization remains parallel to the surface of the thin film for the examined class of probes.

Acknowledgments

I would like to thank Prof. Henryk Szymczak from the Institute of Physics of the Polish Academy of Sciences, who proposed the subject of this paper, stimulated my work on the problem and served me with friendly advice. I also wish to thank Polish Children's Fund, which helped me to develop my skills and knowledge in physics by inviting me to take part in Research Workshop in Physics held in the Institute of Physics of the Polish Academy of Sciences in Warsaw.

Bibliography

1

M.H. Kryder, J. Appl. Phys. 57, 3913 (1985)

2

J.W. Smits, S.B. Luitjens, F.J.A. den Broeder, J. Appl. Phys. 55, 2260 (1984)

3

P. Bruno, J. Appl. Phys. 64, 3153 (1988)

4

Ch. Kittel, Rev. Mod. Phys. 21, 558 (1949)

5

Y. Yafet, E.M. Gyorgy, L.R. Walker, J. Appl. Phys. 60, 4236 (1986)

This document was generated using the LaTeX2HTML translator Version 2K.1beta (1.47)
Copyright © 1993, 1994, 1995, 1996, Nikos Drakos, Computer Based Learning Unit, University of Leeds.
Copyright © 1997, 1998, 1999, Ross Moore, Mathematics Department, Macquarie University, Sydney.